The Effects of Subthalamic Nucleus Deep Brain Stimulation and Retention Delay on Memory-Guided Reaching Performance in People with Parkinson’s Disease
Abstract
Background:
Subthalamic nucleus deep brain stimulation (STN-DBS) improves intensive aspects of movement (velocity) in people with Parkinson’s disease (PD) but impairs the more cognitively demanding coordinative aspects of movement (error). We extended these findings by evaluating STN-DBS induced changes in intensive and coordinative aspects of movement during a memory-guided reaching task with varying retention delays.
Objective:
We evaluated the effect of STN-DBS on motor control during a memory-guided reaching task with short and long retention delays in participants with PD and compared performance to healthy controls (HC).
Methods:
Eleven participants with PD completed the motor section of the Movement Disorder Society Unified Parkinson’s Disease Rating Scale (MDS-UPDRS III) and performed a memory-guided reaching task under four different STN-DBS conditions (DBS-OFF, DBS-RIGHT, DBS-LEFT, and DBS-BOTH) and two retention delays (0.5 s and 5 s). An additional 13 HC completed the memory-guided reaching task.
Results:
Unilateral and bilateral STN-DBS improved the MDS-UPDRS III scores. In the memory-guided reaching task, both unilateral and bilateral STN-DBS increased the intensive aspects of movement (amplitude and velocity) in the direction toward HC but impaired coordinative aspects of movement (error) away from the HC. Furthermore, movement time was decreased but reaction time was unaffected by STN-DBS. Shorter retention delays increased amplitude and velocity, decreased movement times, and decreased error, but increased reaction times in the participants with PD. There were no interactions between STN-DBS condition and retention delay.
Conclusion:
STN-DBS may affect cognitive-motor functioning by altering activity throughout cortico-basal ganglia networks and the oscillatory activity subserving them.
INTRODUCTION
Parkinson’s disease (PD) is a neurodegenerative disorder that affects nearly one million people in the United States [1], with 1% of the population over 60 years old receiving a diagnosis [2, 3]. People with PD experience a range of motor symptoms including tremor, rigidity, and bradykinesia, as well as impairments to gait, balance, and posture. These motor symptoms are a result of degeneration of mesencephalic dopaminergic cells within the substantia nigra pars compacta of the basal ganglia. Subthalamic nucleus deep brain stimulation (STN-DBS) is a therapeutic intervention that effectively improves intensive aspects of movement, such as amplitude and velocity [4, 5]. However, STN-DBS can lead to contrasting effects on inhibitory control, sometimes improving motor inhibition [6–10], and sometimes impairing it or other executive functions [11–15]. STN-DBS can also have negative effects on coordinative aspects of movement [16], leading to unfavorable side effects of the treatment.
Intensive aspects of movement relate to how energetically demanding and forceful a movement is, and they have only one dimension of control, making them less complex and cognitively demanding than coordinative aspects of movement [16–19]. It has been well-established that people with PD illustrate altered beta and gamma oscillatory activity within the basal ganglia and sensorimotor cortices [20–22]. Intensive aspects of movement, such as amplitude and velocity, may be improved with STN-DBS by modifying the aberrant beta and gamma oscillatory activity within the basal ganglia and sensorimotor cortices to ultimately facilitate motor output [23–27]. Coordinative aspects of movement are more complex, with multiple dimensions of processing required for proper execution, such as reaching toward a doorknob or typing on a keyboard. These movements require coordination between different components of movement and sensorimotor integration [16, 18, 19]. Therefore, coordinative movements require a higher level of cognitive control than intensive aspects of movement and are thought to be more difficult to appropriately manipulate with the application of STN-DBS [16, 28]. High frequency STN-DBS may disrupt certain aspects of cognition and coordinative aspects of movement by altering oscillatory activity within the delta, theta, and beta bands that is critical for communication between the STN and the cortex [22, 29].
Memory-guided reaching tasks provide a viable means to assess both intensive and coordinative aspects of movement. During memory-guided reaching tasks, participants are required to encode a target location using sensory input (i.e., central vision or peripheral vision, and/or proprioception) and then maintain that target location for a set temporal interval. Next, the participant is required to prepare the reaching movement by transforming the spatial information about the memorized target into the appropriate movement plan [30, 31]. Finally, the movement is executed while utilizing both feedforward and feedback motor control processes [30, 31]. During a memory-guided reaching task, velocity and amplitude of the movement can be used as measures to assess the intensive aspects of motor control [17]. At the same time, the accuracy of the movement depends on successful encoding of the target location and the ability to retain it in memory, as well as coordinating the sensory and motor transformations necessary to properly plan and execute the movement [16]. Thus, reaching accuracy/error provides a measurement to assess coordinative motor control. Additionally, reaction time and movement time are valuable outcome measures that can be obtained from memory-guided reaching tasks and used to further assess both cognitive and motor control processing.
Both unilateral and bilateral STN-DBS have been shown to impair coordinative aspects of movement (i.e., decrease accuracy) [14, 16], especially when cognitive demand is increased. Previously, we confirmed this hypothesis by using a sequential memory-guided reaching task in which the spatial load was increased (i.e., three targets had to be memorized) [16]. Both bilateral and unilateral STN-DBS, relative to off STN-DBS, improved intensive aspects of movement (velocity) but concurrently decreased movement accuracy [16]. An alternative method to increase cognitive demand is by increasing the temporal load—the duration for which spatial information about the target location must be retained in memory (i.e., the retention delay) [32]. The retention delay can affect certain motor outcomes of the ensuing movement. For example, reaction time is usually shorter when the target is held in memory for a longer period of time [33]. Increased retention delay can also result in reduced accuracy of movements during memory-guided reaching in both persons with PD and healthy controls (HC) [34]. However, the effects of STN-DBS on intensive and coordinative aspects of movement when the retention delay is manipulated have not been investigated. Determining the effects of retention delay on intensive and coordinative aspects of movement in people with PD, and whether STN-DBS exacerbates these effects, is imperative to developing STN-DBS parameters that minimize adverse effects on coordinative aspects of movement while maintaining the beneficial effects on intensive aspects of movement. Furthermore, a HC group comparison can help to establish whether the changes in movement control induced by STN-DBS are in the direction of what is seen in a normative population.
The goal of this investigation was to further probe the effects of STN-DBS on intensive and coordinative aspects of movement by manipulating the retention delay during a memory-guided reaching task in a group of participants with advanced PD. Based on our previous work and the work of others, we hypothesized that both unilateral and bilateral STN-DBS would improve intensive aspects of movement (amplitude and velocity) toward what is seen in HC, with bilateral STN-DBS having the greatest effect. We predicted the effect of unilateral STN-DBS would be greater on the side contralateral to the extremity the task was performed with. As the task was performed with the right arm, we predicted that unilateral STN-DBS on the left side would increase amplitude and velocity more than STN-DBS on the right side. We also hypothesized that both unilateral and bilateral STN-DBS would impair coordinative aspects of movement (error) away from what is seen in HC, with bilateral STN-DBS having the greatest effect. We predicted STN-DBS on the left side would increase error more than STN-DBS on the right side. We also determined whether STN-DBS had an effect on reaction time and movement time. Furthermore, we evaluated whether retention delay had an effect on intensive and coordinative aspects of movement and determined whether these effects would be greater with STN-DBS on compared to STN-DBS off (i.e., if an interaction would occur between retention delay and STN-DBS condition). Finally, we utilized a HC group to determine whether the effects on intensive and coordinative aspects of movement induced by STN-DBS were in the direction of what was seen in a normative population. We discuss our findings in the context of the oscillation model of dysfunction in PD and how changes induced by STN-DBS might improve intensive aspects but impair coordinative aspects of movement.
METHODS
Participants
This study was conducted with approval from the Rush University Medical Center and Northwestern University Institutional Review Boards, and informed consent was obtained from all participants. The study was done in accord with the Helsinki Declaration of 1975. We recruited 14 participants with advanced PD and bilateral high frequency STN-DBS. Participants with PD were recruited from the Rush University Medical Center Movement Disorders Clinic and Northwestern University. All participants were examined by a movement disorders neurologist and included in the study if they: met the UK PD Society brain bank clinical diagnostic criteria for PD [35, 36], had successful response to STN-DBS surgery, were able to understand and perform the experimental tasks, had normal or corrected visual acuity, presented no eye movement abnormalities such as double vision and/or blepharospasm, had no other neurological comorbidities, and had no orthopedic issues that could preclude completing experimental tasks. In addition, electrode placement in the sensorimotor area of the STN was confirmed by microelectrode recording from multiple kinesthetic cells. Three participants with PD were unable to complete testing while OFF stimulation and were excluded from the final analysis. This left 11 participants with PD (Mean age = 66.64±3.17 years, Females = 0) in the final analysis.
An additional cohort of 16 HC participants were recruited. Healthy controls were assessed by one of the study investigators. They were included in the study if they had no reported history of neurological disorders, had a score of≤6 on the Movement Disorder Society Unified Parkinson’s Disease Rating Scale (MDS-UPDRS III) [31] and a Montreal Cognitive Assessment (MoCA)≥23. The cut-off of≤6 for HC was determined from the mean MDS-UPDRS III (+2 standard deviations) from HC (n = 196) who were part of the Parkinson’s Progression Markers Initiative database [37]. A MoCA of < 23 is considered in the range of cognitive impairment [38]. Apart from these three criteria, HC met the same inclusion/exclusion criteria as those with PD. Three HC participants were excluded from our statistical analyses: one had a MoCA score of 18, one had an MDS-UPDRS III score of 12, and one was unable to attend to the instructional set and did not provide sufficient data to be included in the final analysis. Thus, 13 HC participants were used in the final analysis (Mean age = 65.23±4.34 years, Females = 2, MoCA = 27.38).
All participants were right-handed and used their right hand to complete the memory-guided reaching task. The Edinburgh Handedness Inventory was used to confirm right hand dominance [39]. For further demographic information on the participants with PD, see Table 1. For the stimulation settings for each of the participants with PD, see Table 2.
Table 1
Patient demographics
| Parkinson’s disease | |
| Sex (M/F) | 11/0 |
| Age (mean±SD, y) | 66.64±3.17 |
| MoCA (mean±SD) | 27.00±2.00 |
| MDS-UPDRS Part III, DBS-OFF while Off meds (mean±SD) | 50.55±14.27 |
| MDS-UPDRS Part III, DBS-RIGHT while Off meds (mean±SD) | 38.09±10.29 |
| MDS-UPDRS Part III, DBS-LEFT while Off meds (mean±SD) | 37.64±12.62 |
| MDS-UPDRS Part III, DBS-BOTH while Off Meds (mean±SD) | 19.70±7.28 |
| Months Post Surgery (mean±SD) | 8.27±1.74 |
| LEDD (mg±SD) | 405.45±288.92 |
Table 2
Patient stimulation settings
| Voltage (left/right) | Frequency (left/right) | Pulse width (left/right) |
| 3.0 volts/4.0 volts | 130 Hz/130 Hz | 60 μs /60 μs |
| 2.4 mA/2.2 mA | 130 Hz/130 Hz | 60μs /60 μs |
| 3.0 volts/3.2 volts | 125 Hz/125 Hz | 60 μs /60 μs |
| 2.5 volts/2.9 volts | 130 Hz/130 Hz | 60 μs /60 μs |
| 2.9 mA/2.0 mA | 160 Hz/130 Hz | 90 μs /60 μs |
| 2.4 mA/3.2 mA | 130 Hz/130 Hz | 60 μs /60 μs |
| 2.9 mA/2.9 mA | 130 Hz/130 Hz | 60 μs /60 μs |
| 3.0 mA/3.1 mA | 130 Hz/130 Hz | 60 μs /60 μs |
| 2.1 mA/2.7 mA | 130 Hz/130 Hz | 60 μs /60μs |
| 3.8 mA/2.8 mA | 130 Hz/130 Hz | 60 μs /60 μs |
| 3.6 mA/2.5 mA | 130 Hz/180 Hz | 60μs /60 μs |
Each row denotes the stimulation settings for a different participant.
Experimental conditions
Data collection took place over five days. On the first day of testing, participants with PD arrived while on treatment and were consented and acclimatized to the laboratory and experimental tasks. Participants performed the experimental task under four different stimulation conditions over the next four days (one stimulation condition per day): both stimulators off (DBS-OFF), right unilateral on (DBS-RIGHT), left unilateral on (DBS-LEFT) and both on (DBS-BOTH). Stimulators were turned off three hours prior to testing, which provided a practically feasible washout period and sufficient time for the patient’s condition to stabilize [40]. Participants with PD refrained from PD medications at least 12 hours prior to testing. The order of testing conditions was randomized. On each testing day, testing commenced at 9 am and ended no later than 1 pm. Each day began with the administration of the MDS-UPDRS III followed by the practice and execution of 6 different experimental tasks. Breaks and light snacks were provided between each experimental task. The memory-guided reaching task was always administered during the 1st half of each testing session. Only the findings from the memory-guided reaching tasks will be reported in this paper.
Instrumentation for the memory-guided reaching task
The memory-guided reaching task was conducted in a completely darkened room. Participants were seated upright on an adjustable chair, with their chin on a chin rest to minimize head movement. Head and finger movements were captured with a 3D motion capture system (Northern Digital,Waterloo, Canada). Eye movements were captured at 500 Hz with a head-mounted video-based eye-tracking system (SR Research, Ottawa, Canada). An active infrared emitting diode was taped to the participant’s index finger to track finger movements (Northern Digital, Waterloo, Canada).
One target was presented to the participants using a five-degree of freedom robot arm (Thermo CRS, Burlington, Ontario, Canada). A central fixation light (3 mm green LED, 70 mcd) was situated 42 cm away from the subject at 0° visual angle attached to a fixation stand. This served as the starting point for the subject’s reaching motion. The robot presented the target (3 mm green LED, 70 mcd) in a plane that was 42 cm from the chin rest.
Head, finger, and robot movements were synchronized and stored using the Motion Monitor system (TheMotionMonitor, Innovative Sports Training, Chicago, IL, USA).
Protocol
Each testing session began with the administration of the MDS-UPDRS III [41] with the memory-guided reaching task performed afterwards. The memory-guided reaching task began with subjects fixating on the central fixation light (0° visual angle) for 2 – 3 s with their finger on a fixation stand. This was done to ensure that the finger and the eye focal point were at the same location in the horizontal plane. With the central fixation still lit, the robotic arm flashed one target in the participants’ visual field for 0.05 s. One of two targets were presented, one at 10° (linear distance of 0.074 m) or one at 15° (linear distance of 0.113 m) visual angle from central fixation. Target location was randomized. During the encoding phase, participants were asked to only use peripheral vision to remember the target’s location. After either a 0.5 s or 5 s retention delay, the central fixation light was extinguished. This served as the cue for participants to initiate reaching to the remembered target. Participants were instructed to “reach as quickly as you can and make one smooth movement out to the remembered target.” When the reaching movement was completed, participants returned their finger to the central fixation stand, which was illuminated by a flashlight by one of the experimenters. The experimental paradigm is illustrated in Fig. 1.
Fig. 1
Memory-guided reaching task. Illustration of the experimental task. The memory-guided reaching task was performed with the right hand, and reaches were performed in a 3D space. The quadrilateral depicts the plane in which the fixation and targets were presented. Variation of temporal load occurred during Retention Delay and was either 0.5 s or 5 s.
Participants performed one block of 40 trials, in which 20 trials were performed with the short target (10°, 0.074 m) and 20 were performed with the long target (15°, 0.113 m). For the purpose of this study, we were assessing the effects of STN-DBS condition and retention delay, and not the effect of target distance. Thus, we chose to only analyze trials with a longer distance, as these trials were likely more difficult and, thus, more sensitive to elicit differences in motor control. Within those 20 trials, 10 had a 0.5 s delay and 10 had a 5 s delay. Prior to test trials, all participants performed at least five practice trials until they could perform the task correctly. Practice trials were not analyzed.
Data analysis
The data were analyzed using a custom MATLAB script (MATLAB 2021b, Natick, United States). A 20 Hz low-pass 2nd order, zero-phase Butterworth filter was applied to the finger position signals. The filtered position data was then differentiated to calculate velocity. The amplitude (total distance moved during the reach) and peak velocity during the reach were used as the outcome measures evaluating intensive aspects of movement.
Reaching error was used as the outcome measure to evaluate coordinative aspects of movement. Furthermore, reaction time and movement time were assessed to further determine whether STN-DBS impacts cognitive and motor processing. The following procedure was used to determine these measures. First, finger endpoints to the target were visually marked. From the visually determined finger endpoints, an algorithm searched backwards to determine the first peak in the finger velocity profile. This peak was associated with the finger movement that brought the finger to the target location. Thus, time points corresponding to the peak were established. Next, from this peak, the algorithm searched forwards to detect the first time point when finger velocity went below 5% of peak velocity and stayed below this threshold for 0.2 s [42]. The first time point when this condition was met was designated as the finger endpoint. Next, from the first peak, the algorithm searched backwards to detect the first time point when finger velocity went below 5% of peak velocity and stayed below this threshold for 0.2 s. The first time point when this condition was met was designated as the onset of the finger movement. The difference between this point in time and the cue to initiate the movement was defined as the finger reaction time, and the difference between the onset of the finger movement and the time corresponding to the finger endpoint was defined as the movement time. The locations of the finger endpoints were then used to calculate error by subtracting the values of these locations from the values of the corresponding target locations. Error was calculated in all three dimensions for the finger endpoints. The magnitude of finger end-point error was calculated using the following equation:
Finger end point error magnitude =
(1)
For the memory-guided reaching task, outlier trials were removed if they had reaction times < 0.2 s or > 2.5 s, peak velocity > 2 m/s, and error > 0.2 m. In addition, those trials where the participant looked at the target were deemed invalid and were not analyzed.
Statistical analysis
A mixed effect regression model was used to assess the effect of STN-DBS on the MDS-UPDRS III, with STN-DBS condition (DBS-OFF, DBS-LEFT, DBS-RIGHT, and DBS-BOTH) being the fixed effect and participant being the random effect. Mixed effect regression models were then utilized to assess the effect of STN-DBS and retention delay on memory-guided reaching performance. The fixed effects used were stimulation condition and retention delay (0.5 s and 5 s). The random effect was participant. Amplitude was used as a time-varying covariate in the velocity analysis, given the known positive relationship between movement amplitude and velocity [43]. In addition, for the finger endpoint error outcomes, finger velocity was used as time varying covariates in our respective mixed effect regression models. This was done because first, there is a well-known speed accuracy trade-off, i.e., faster movements are likely to be associated with greater errors [16, 43, 44]. Second, it is also established that STN-DBS is very effective at improving movement speed. Therefore, we wanted to control for any differences in velocity as a consequence of stimulation condition that might contribute to increases in error. If the omnibus F-test was significant for condition, then post-hoc pairwise comparisons were performed using the Tukey Kramer method to correct for multiple comparisons.
Separate mixed effect regression models were performed to assess the difference between the participants with PD for each STN-DBS condition and HC for each outcome measure, with group (HC and PD) being the fixed effect and participant being the random effect. Finally, mixed effect regression models were used to determine the effect of retention delay in the HC participants, with retention delay (0.5 s and 5 s) as the fixed effect and participant as the random effect.
In addition, Bayes Factors (BF10) were computed for each of the statistical comparisons. The BF10 provides a ratio of how likely the alternative hypotheses are in comparison with the null hypotheses, and we used these to further support our findings.
All statistical analyses were performed using SAS™ (version 9.4; SAS Institute, Cary, NC). All statistical tests were two-sided, critical alpha was 0.05, and p values associated with all pairwise comparisons were corrected using the Tukey-Kramer method. Results are reported as the mean±standard deviation.
RESULTS
MDS-UPDRS III
There was a main effect of STN-DBS condition for the MDS-UPDRS III (F3,30 = 27.77, p < 0.001). Post-hoc analysis revealed that DBS-BOTH had lower scores than the DBS-LEFT (DBS-BOTH = 19.55±8.17, DBS-LEFT = 37.64±12.62, t(30) = – 5.28, Cohen’s D = 1.34, p < 0.001, BF10 > 50), DBS-RIGHT (DBS-BOTH = 19.55±8.17, DBS-RIGHT = 38.09±10.29, t(30) = – 5.42, Cohen’s D = 1.71, p < 0.001, BF10 > 50), and DBS-OFF (DBS-BOTH = 19.55±8.17, DBS-OFF = 50.55±14.27, t(30) = – 9.05, Cohen’s D = 1.94, p < 0.001, BF10 >50) conditions. The DBS-LEFT condition had lower scores than DBS-OFF (DBS-LEFT = 37.64±12.62, DBS-OFF = 50.55±14.27, t(30) = – 3.77, Cohen’s D = 1.28, p = 0.004, BF10 > 50). The DBS-RIGHT condition had lower scores than DBS-OFF (DBS-RIGHT = 38.09±10.29, DBS-OFF = 50.55±14.27, t(30) = – 3.64, Cohen’s D = 1.31, p = 0.005, BF10 = 38.7). DBS-RIGHT and DBS-LEFT had similar scores (DBS-RIGHT = 38.09±10.29, DBS-LEFT = 37.64±12.62, t(30) = –0.13, Cohen’s D = 0.05 p = 0.999, BF10 = 0.58). For a full list of statistical values, see Table 3.
Table 3
Mixed Effect Regression: Effect of STN-DBS condition on MDS-UPDRS III
| MDS-UPDRS III | |
| Condition | p < 0.001* F = 27.77 |
| BOTH vs. LEFT | p < 0.001* t = – 5.28 Diff = – 18.091 BF10 > 50 Cohen’s D = 1.34 |
| BOTH vs. RIGHT | p < 0.001* t = – 5.42 Diff = – 18.546 BF10 > 50 Cohen’s D = 1.71 |
| BOTH vs. OFF | p < 0.001* t = – 9.05 Diff = – 31.000 BF10 > 50 Cohen’s D = 1.94 |
| LEFT vs. RIGHT | p = 0.999 t = – 0.13 Diff = – 0.455 BF10 = 0.58 Cohen’s D = 0.05 |
| LEFT vs. OFF | p = 0.004* t = – 3.77 Diff = – 12.909 BF10 > 50 Cohen’s D = 1.28 |
| RIGHT vs. OFF | p = 0.005* t = – 3.64 Diff = – 12.455 BF10 = 38.7 Cohen’s D = 1.31 |
*p < 0.05.
Amplitude
The number of trials we used per condition in the memory-guided reaching task was 10. On average, we ended up with 8.5 trials per participant, per stimulation condition, per delay.
There was no interaction between STN-DBS condition and retention delay for amplitude (F(3, 731) = 2.03, p = 0.108). There was a main effect of STN-DBS condition for amplitude (F(3, 731) = 23.48, p < 0.001). Post-hoc analysis revealed that DBS-BOTH had higher amplitude than the DBS-LEFT (DBS-BOTH = 0.156±0.042 m, DBS-LEFT = 0.147±0.146 m, t(731) = 4.27, Cohen’s D = 0.699, p < 0.001, BF10 > 50), DBS-RIGHT (DBS-BOTH = 0.156±0.042 m, DBS-RIGHT = 0.143±0.043 m, t(731) = 5.58, Cohen’s D = 0.812, p < 0.001, BF10 > 50), and DBS-OFF (DBS-BOTH = 0.156±0.042 m, DBS-OFF = 0.138±0.053 m, t(731) = 8.15, Cohen’s D = 0.803, p < 0.001, BF10 > 50) conditions. DBS-LEFT also had higher amplitude than DBS-OFF (DBS-LEFT = 0.147±0.046 m, DBS-OFF = 0.138±0.053 m, t(731) = 4.07, Cohen’s D = 0.602, p < 0.001, BF10 > 50). No other conditions were different from each other (Fig. 2A).
Fig. 2
A) Estimated mean (±standard error) of amplitude for DBS-OFF, RIGHT, LEFT, BOTH, and the HC (left to right). DBS-LEFT and DBS-BOTH increased reaching amplitude relative to DBS-OFF (p < 0.001 for both). DBS-BOTH also increased amplitude relative to DBS-RIGHT and DBS-LEFT (p < 0.001 for both). The bars above the graph denote the significant differences in amplitude between STN-DBS conditions. B) The effect of retention delay on amplitude in the participants with PD and HC. Amplitude was significantly increased during the 0.5 s delay relative to the 5 s delay in participants with PD (p < 0.001). The bars above the graph denote the significant differences in amplitude between the short and long retention delays.
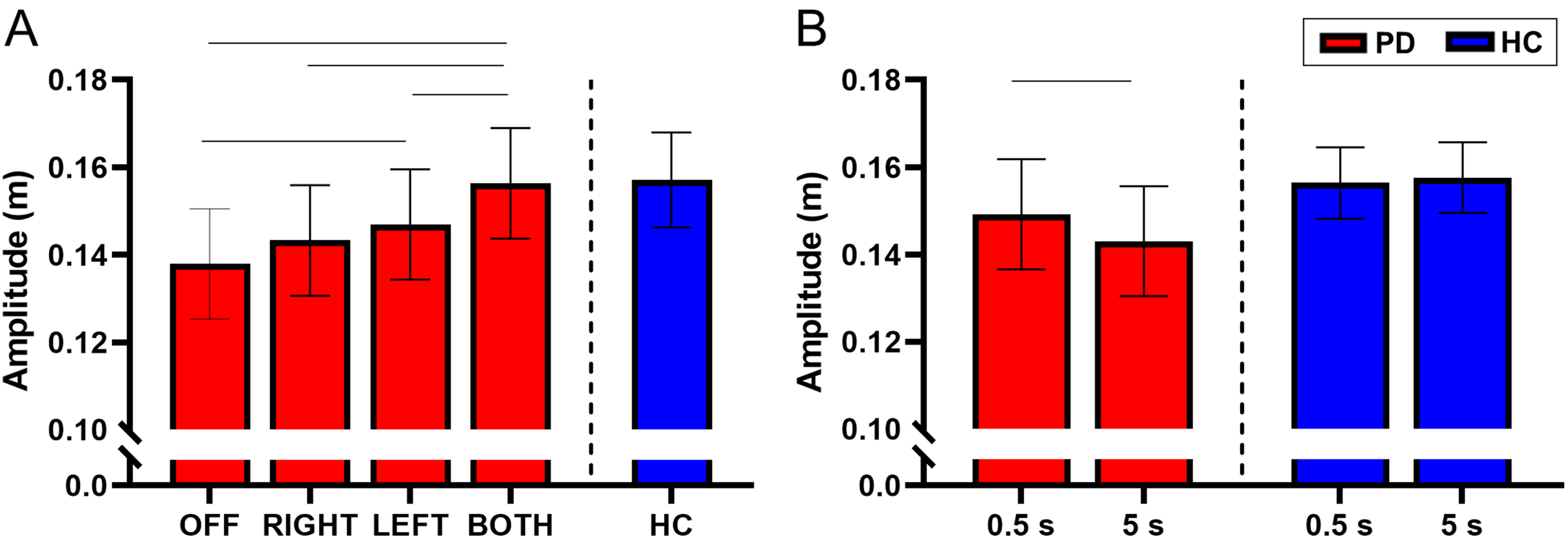
There was a main effect of retention delay on amplitude in the participants with PD. The amplitude was lower during the long delay compared with the short delay (short delay = 0.149±0.046 m, long delay = 0.143±0.047 m, F(1, 731) = 14.91, Cohen’s D = 0.516, p < 0.001, BF10 = 8.57) (Fig. 2B). For a full list of statistical values, see Table 4.
Table 4
Mixed Effect Regression: Effect of STN-DBS condition and retention delay on memory-guided reaching outcomes
| Amplitude (m) | Velocity (m/s) | Error (m) | Reaction Time (s) | Movement Time (s) | |
| Condition | p < 0.001* | p < 0.001* | p < 0.001* | p = 0.746 | p < 0.001* |
| F = 23.48 | F = 12.90 | F = 13.84 | F = 0.41 | F = 12.92 | |
| BOTH vs. LEFT | p < 0.001* | p = 0.019* | p = 0.117 | p = 0.982 | p = 0.608 |
| t = 4.27 | t = 2.92 | t = 2.23 | t = 0.37 | t = – 1.23 | |
| Diff = 0.009 | Diff = 0.037 | Diff = 0.004 | Diff = 0.007 | Diff = – 0.027 | |
| BF10 > 50 | BF10 > 50 | BF10 = 1.34 | BF10 = 0.60 | BF10 = 0.27 | |
| Cohen’s D = 0.699 | Cohen’s D = 0.226 | Cohen’s D = 0.275 | Cohen’s D = 0.098 | Cohen’s D = 0.133 | |
| BOTH vs. RIGHT | p < 0.001* | p < 0.001* | p < 0.001* | p = 0.904 | p < 0.001* |
| t = 5.58 | t = 5.40 | t = 4.37 | t = – 0.68 | t = – 4.04 | |
| Diff = 0.013 | Diff = 0.074 | Diff = 0.009 | Diff = – 0.013 | Diff = – 0.094 | |
| BF10 > 50 | BF10 > 50 | BF10 > 50 | BF10 = 0.60 | BF10 > 50 | |
| Cohen’s D = 0.812 | Cohen’s D = 0.481 | Cohen’s D = 0.496 | Cohen’s D = 0.071 | Cohen’s D = 0.479 | |
| BOTH vs. OFF | p < 0.001* | p < 0.001* | p < 0.001* | p = 0.998 | p < 0.001* |
| t = 8.15 | t = 5.27 | t = 6.05 | t = 0.19 | t = – 5.49 | |
| Diff = 0.018 | Diff = 0.071 | Diff = 0.013 | Diff = 0.003 | Diff = 0.124 | |
| BF10 > 50 | BF10 > 50 | BF10 > 50 | BF10 = 0.56 | BF10 > 50 | |
| Cohen’s D = 0.803 | Cohen’s D = 0.292 | Cohen’s D = 0.566 | Cohen’s D = 0.014 | Cohen’s D = 0.564 | |
| LEFT vs. RIGHT | p = 0.383 | p = 0.029* | p = 0.076 | p = 0.718 | p = 0.018* |
| t = 1.59 | t = 2.77 | t = 2.41 | t = – 1.05 | t = – 2.94 | |
| Diff = 0.004 | Diff = 0.037 | Diff = 0.005 | Diff = – 0.019 | Diff = – 0.067 | |
| BF10 = 0.72 | BF10 = 11.63 | BF10 = 3.96 | BF10 = 0.78 | BF10 = 13.1 | |
| Cohen’s D = 0.238 | Cohen’s D = 0.321 | Cohen’s D = 0.224 | Cohen’s D = 0.192 | Cohen’s D = 0.378 | |
| LEFT vs. OFF | p < 0.001* | p = 0.043* | p < 0.001* | p = 0.998 | p < 0.001* |
| t = 4.07 | t = 2.63 | t = 4.10 | t = – 0.18 | t = – 4.36 | |
| Diff = 0.009 | Diff = 0.034 | Diff = 0.008 | Diff = – 0.003 | Diff = 0.097 | |
| BF10 > 50 | BF10 = 11.63 | BF10 > 50 | BF10 = 0.59 | BF10 > 50 | |
| Cohen’s D = 0.602 | Cohen’s D = 0.148 | Cohen’s D = 0.500 | Cohen’s D = 0.082 | Cohen’s D = 0.470 | |
| RIGHT vs. OFF | p = 0.101 | p = 0.998 | p = 0.406 | p = 0.826 | p = 0.587 |
| t = 2.29 | t = – 0.19 | t = 1.55 | t = 0.86 | t = – 1.26 | |
| Diff = 0.005 | Diff = – 0.003 | Diff = 0.003 | Diff = 0.016 | Diff = 0.030 | |
| BF10 = 8.57 | BF10 = 0.15 | BF10 = 1.34 | BF10 = 0.6 | BF10 = 0.49 | |
| Cohen’s D = 0.248 | Cohen’s D = 0.018 | Cohen’s D = 0.162 | Cohen’s D = 0.111 | Cohen’s D = 0.149 | |
| Delay | p < 0.001* | p = 0.030* | p = 0.005* | p < 0.001* | p < 0.001* |
| F = 14.91 | F = 4.74 | F = 7.85 | F = 110.69 | F = 27.07 | |
| Diff = – 0.006 | Diff = – 0.020 | Diff = 0.004 | Diff = – 0.135 | Diff = 0.083 | |
| BF10 = 8.57 | BF10 = 1.45 | BF10 > 50 | BF10 > 50 | BF10 > 50 | |
| Cohen’s D = 0.516 | Cohen’s D = 0.402 | Cohen’s D = 0.456 | Cohen’s D = 0.930 | Cohen’s D = 0.445 |
*p < 0.05.
Compared with the HC, the participants with PD did not have a statistically significant difference in the amplitude of movement during each of the stimulation conditions (p’s > 0.05) (Fig. 2A and Table 5). However, inspection of the data in Fig. 2A does show a trend in which the amplitude increases across stimulation conditions and approaches values of HC. The lack of statistical significance between participants with PD and HC may be due to lack of experimental power for the between group comparison of PD and HC.
Table 5
Mixed Effect Regression: PD vs. HC group comparison
| Amplitude (m) | Velocity (m/s) | Error (m) | Reaction Time (s) | Movement Time (s) | |
| OFF vs. HC | p = 0.206 | p = 0.013* | p = 0.383 | p = 0.076 | p = 0.006* |
| t = – 1.27 | t = – 2.48 | t = 0.87 | t = 1.78 | t = 2.77 | |
| Diff = – 0.020 | Diff = – 0.295 | Diff = 0.012 | Diff = 0.104 | Diff = 0.317 | |
| BF10 = 0.91 | BF10 = 4.60 | BF10 = 0.50 | BF10 = 1.36 | BF10 = 14.35 | |
| Cohen’s D = 0.497 | Cohen’s D = 0.983 | Cohen’s D = 0.359 | Cohen’s D = 0.685 | Cohen’s D = 1.104 | |
| RIGHT vs. HC | p = 0.323 | p = 0.009* | p = 0.158 | p = 0.043* | p = 0.011* |
| t = 0.99 | t = – 2.61 | t = 1.41 | t = 2.04 | t = 2.55 | |
| Diff = – 0.014 | Diff = – 0.306 | Diff = 0.019 | Diff = 0.117 | Diff = 0.285 | |
| BF10 = 0.67 | BF10 = 6.59 | BF10 = 1.91 | BF10 = 2.22 | BF10 = 7.37 | |
| Cohen’s D = 0.397 | Cohen’s D = 0.944 | Cohen’s D = 0.618 | Cohen’s D = 0.797 | Cohen’s D = 1.027 | |
| LEFT vs. HC | p = 0.509 | p = 0.026* | p = 0.108 | p = 0.114 | p = 0.039* |
| t = – 0.66 | t = – 2.23 | t = 1.61 | t = 1.58 | t = 2.07 | |
| Diff = – 0.010 | Diff = – 0.261 | Diff = 0.023 | Diff = 0.096 | Diff = 0.225 | |
| BF10 = 0.69 | BF10 = 2.81 | BF10 = 1.91 | BF10 = 1.36 | BF10 = 3.42 | |
| Cohen’s D = 0.259 | Cohen’s D = 0.824 | Cohen’s D = 0.670 | Cohen’s D = 0.615 | Cohen’s D = 0.816 | |
| BOTH vs. HC | p = 0.922 | p = 0.053 | p = 0.030* | p = 0.054 | p = 0.075 |
| t = – 0.10 | t = – 1.94 | t = 2.17 | t = 1.93 | t = 1.78 | |
| Diff = – 0.001 | Diff = – 0.229 | Diff = 0.030 | Diff = 0.110 | Diff = 0.200 | |
| BF10 = 0.45 | BF10 = 1.81 | BF10 = 18.15 | BF10 = 1.72 | BF10 = 1.72 | |
| Cohen’s D = 0.038 | Cohen’s D = 0.761 | Cohen’s D = 0.924 | Cohen’s D = 0.655 | Cohen’s D = 0.671 |
*p < 0.05.
Within the HC participants, there was no effect of retention delay on amplitude (short delay = 0.156±0.031 m, long delay = 0.158±0.034 m, t(215) = 0.48, Cohen’s D = 0.093, p = 0.634, BF10 = 0.13) (Fig. 2B and Table 6).
Table 6
Mixed Effect Regression: Effect of Retention Delay in HC
| Amplitude (m) | Velocity (m/s) | Error (m) | Reaction Time (s) | Movement Time (s) | |
| Delay | p = 0.634 | p < 0.001* | p = 0.049* | p < 0.001* | p = 0.001* |
| t = 0.48 | t = – 5.23 | t = 1.98 | t = – 9.51 | t = 3.53 | |
| Diff = 0.001 | Diff = – 0.067 | Diff = 0.004 | Diff = – 0.197 | Diff = 0.090 | |
| BF10 = 0.13 | BF10 > 50 | BF10 = 1.41 | BF10 > 50 | BF10 = 17.84 | |
| Cohen’s D = 0.093 | Cohen’s D = 0.765 | Cohen’s D = 0.454 | Cohen’s D = 1.260 | Cohen’s D = 0.870 |
*p < 0.05.
Velocity
There was no interaction between STN-DBS condition and retention delay for velocity (F(3, 730) = 0.55 p = 0.645). There was a main effect of STN-DBS condition for velocity (F(3, 730) = 12.90, p < 0.001). Post hoc analysis revealed that DBS-BOTH had higher velocity than the DBS-LEFT (DBS-BOTH = 0.425±0.154 m/s, DBS-LEFT = 0.388±0.165 m/s, t(730) = 2.92, Cohen’s D = 0.226, p = 0.019, BF10 > 50), DBS-RIGHT (DBS-BOTH = 0.425±0.154 m/s, DBS-RIGHT = 0.352±0.124 m/s, t(730) = 5.40, Cohen’s D = 0.481, p < 0.001, BF10 > 50), and DBS-OFF (DBS-BOTH = 0.425±0.154 m/s, DBS-OFF = 0.354±0.186 m/s, t(730) = 5.27, Cohen’s D = 0.292, p < 0.001, BF10 > 50) conditions. DBS-LEFT also had higher velocity than DBS-RIGHT (DBS-LEFT = 0.388±0.165 m/s, DBS-RIGHT = 0.352±0.124 m/s, t(730) = 2.77, Cohen’s D = 0.321, p = 0.029, BF10 = 11.63) and DBS-OFF (DBS-LEFT = 0.388±0.165 m/s, DBS-OFF = 0.354±0.186 m/s, t(730) = 2.63, Cohen’s D = 0.148, p = 0.043, BF10 = 11.63). No other conditions were different from each other (Fig. 3A).
Fig. 3
A) Estimated mean (±standard error) of velocity for both delays for DBS-OFF, RIGHT, LEFT, BOTH, and the HC (left to right). DBS-LEFT and DBS-BOTH increased velocity relative to DBS-OFF (p = 0.043 and < 0.001 respectively). DBS-LEFT and DBS-BOTH also increased velocity relative to DBS-RIGHT (p = 0.029 and < 0.001 respectively). DBS-BOTH velocity was greater than DBS-LEFT (p = 0.019). The bars above the graph denote the significant differences in velocity between STN-DBS conditions. B) The effect of retention delay on velocity in the participants with PD and HC. Velocity was significantly increased during the 0.5 s delay relative to the 5 s delay in participants with PD (p = 0.030) and HC (p < 0.001). The bars above the graph denote the significant differences in velocity between the short and long retention delays.
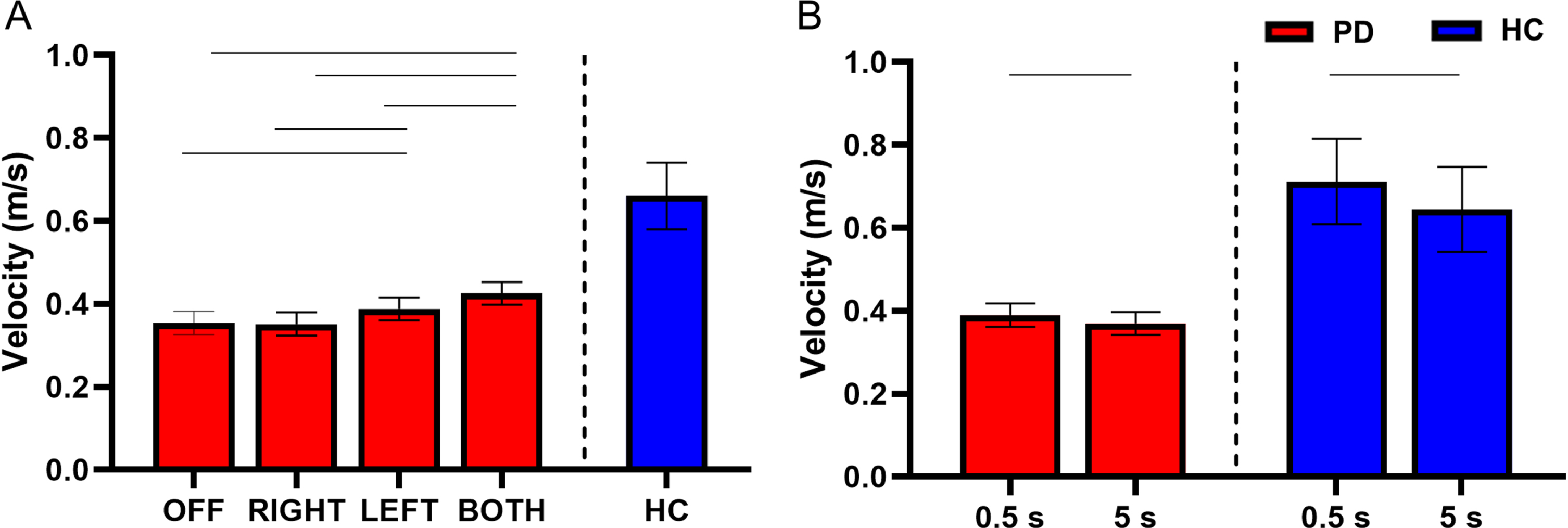
There was a main effect of retention delay on velocity in the participants with PD. Velocity was lower during the long delay in comparison with the short delay (short delay = 0.390±0.172 m/s, long delay = 0.370±0.158 m/s, F(1, 730) = 4.74, Cohen’s D = 0.402, p = 0.030, BF10 = 1.45) (Fig. 3B). For a full list of statistical values, see Table 4.
Compared with the HC, the participants with PD had lower velocity of movement during DBS-LEFT (HC = 0.661±0.281 m/s, DBS-LEFT = 0.400±0.165 m/s, t(412) = – 2.23, Cohen’s D = 0.824, p = 0.026, BF10 = 2.81), DBS-RIGHT (HC = 0.659±0.281 m/s, DBS-RIGHT = 0.353±0.124 m/s, t(376) = – 2.61, Cohen’s D = 0.944, p = 0.009, BF10 = 6.59), and DBS-OFF (HC = 0.660±0.281 m/s, DBS-OFF = 0.365±0.186 m/s, t(384) = – 2.48, Cohen’s D = 0.983, p = 0.013, BF10 = 4.60) (Fig. 3A and Table 5). The difference between DBS-BOTH and HC did not reach significance (DBS-BOTH = 0.439±0.165 m/s, HC = 0.668±0.281 m/s, t(394) = – 1.94, Cohen’s D = 0.761, p = 0.053, BF10 = 1.81).
Within the HC participants, the velocity was lower during the long delay in comparison with the short delay (short delay = 0.712±0.290 m/s, long delay = 0.645±0.274 m/s, t(214) = – 5.23, Cohen’s D = 0.765, p < 0.001, BF10 > 50) (Fig. 3B and Table 6).
Error
There was no interaction between STN-DBS condition and retention delay for error (F(3, 730) = 0.62, p = 0.601). There was a main effect of STN-DBS condition for error (F(3, 730) = 13.84, p < 0.001). Post hoc analysis revealed that DBS-BOTH had higher error than the DBS-RIGHT (DBS-BOTH = 0.073±0.037 m, DBS-RIGHT = 0.064±0.033 m, t(730) = 4.37, Cohen’s D = 0.496, p < 0.001, BF10 > 50) and DBS-OFF (DBS-BOTH = 0.073±0.037 m, DBS-OFF = 0.060±0.041 m, t(730) = 6.05, Cohen’s D = 0.566, p < 0.001, BF10 > 50) conditions. DBS-LEFT also had higher error than DBS-OFF (DBS-LEFT = 0.069±0.041 m, DBS-OFF = 0.060±0.041 m, t(730) = 4.10, Cohen’s D = 0.500, p < 0.001, BF10 > 50). No other conditions were different from each other (Fig. 4A).
Fig. 4
A) Estimated mean (±standard error) of error for both delays for DBS-OFF, RIGHT, LEFT, BOTH, and HC (left to right). DBS-LEFT and DBS-BOTH increased reaching error relative to OFF (p < 0.001 for both). DBS-BOTH also increased error relative to DBS-RIGHT (p < 0.001). The bars above the graph denote the significant differences in error between STN-DBS conditions. B) The effect of retention delay on error for the participants with PD and HC. Error was significantly decreased during the 0.5 s delay relative to the 5 s delay for the participants with PD (p = 0.005) and HC (p = 0.049). The bars above the graph denote the significant differences in error between the short and long retention delays.
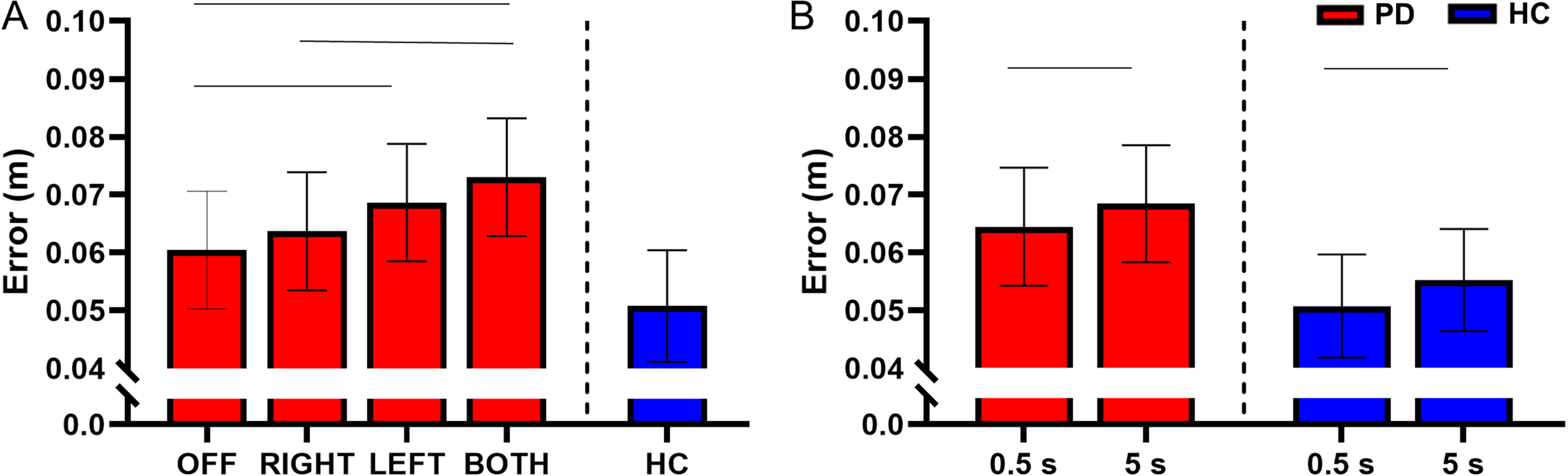
There was a main effect of retention delay for error in the participants with PD. Error was higher during the long delay in comparison with the short delay in the participants with PD (F(1, 731) = 7.85, short delay = 0.064±0.038 m, long delay = 0.068±0.039 m, Cohen’s D = 0.456, p = 0.005, BF10 > 50) (Fig. 4B). For a full list of statistical values, see Table 4.
Compared with the HC, the participants with PD had a significantly higher error during the DBS-BOTH condition (HC = 0.050±0.038 m, DBS-BOTH = 0.080±0.037 m, t(394) = 2.17, Cohen’s D = 0.924, p = 0.030, BF10 = 18.15). No other conditions were significantly different (ps > 0.05) (Fig. 4A and Table 5). However, inspection of the data in Fig. 4A does show a trend in which the error increases across stimulation conditions away from values of HC. The lack of statistical significance between participants with PD and HC may be due to lack of experimental power for the between group comparison of PD and HC.
Within the HC participants, the short retention delay had lower error than the long retention delay (short delay = 0.051±0.030 m, long delay = 0.055±0.032 m, t(214) = 1.98, Cohen’s D = 0.454, p = 0.049, BF10 = 1.41) (Fig. 4B and Table 6).
Reaction time
There was no interaction between retention delay and STN-DBS condition for reaction time (F(3, 731) = 0.85, p = 0.464). There was no main effect of STN-DBS condition for reaction time (F(3, 731) = 0.41, p = 0.746), indicating that the reaction time was similar between all STN-DBS conditions (Fig. 5A).
Fig. 5
A) Estimated mean (±standard error) of reaction time for both delays for DBS-OFF, RIGHT, LEFT, BOTH, and HC (left to right). Stimulation condition had no effect on reaction time. B) The effect of retention delay on reaction time in the participants with PD and HC. Reaction time was significantly longer during the 0.5 s delay relative to the 5 s delay in the participants with PD (p < 0.001) and HC (p < 0.001). The bars above the graph denote the significant differences in reaction time between the short and long retention delays.
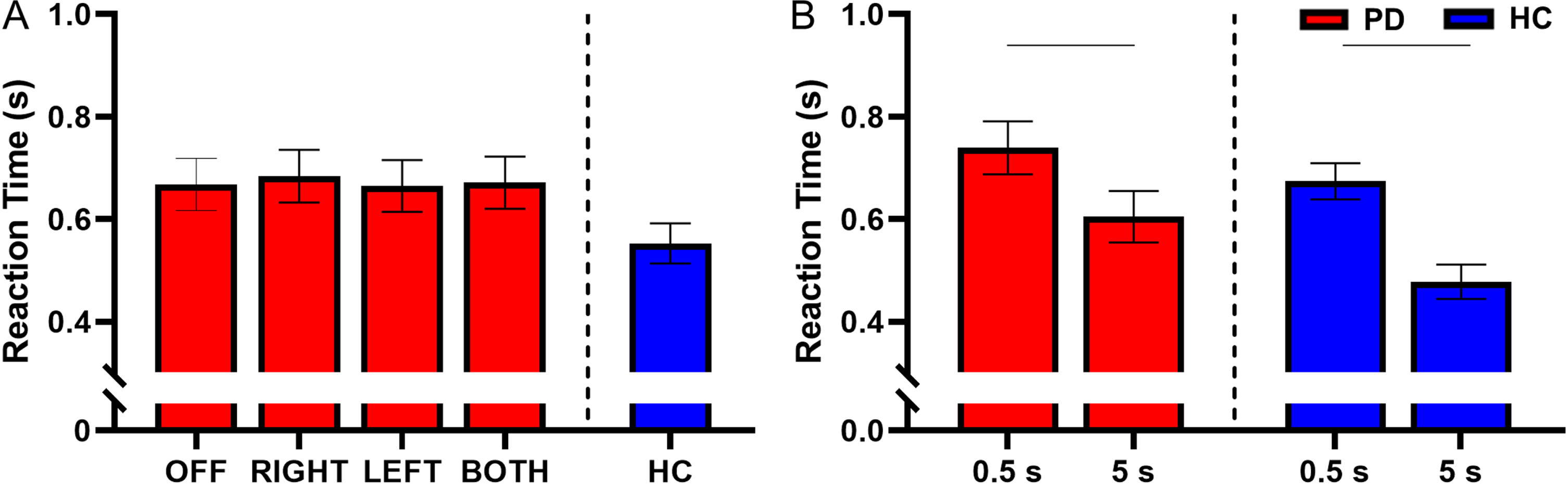
There was a main effect of retention delay for reaction time in participants with PD. Reaction time was shorter during the long delay compared with the short delay (F(1, 731) = 110.69, short delay = 0.739±0.246 s, long delay = 0.604±0.208 s, Cohen’s D = 0.930, p < 0.001, BF10 > 50) (Fig. 5B). For a full list of statistical values, see Table 4.
Compared with the HC, participants with PD had longer reaction times during DBS-RIGHT (HC = 0.553±0.208 s, DBS-RIGHT = 0.670±0.208 s, t(377) = 2.04, Cohen’s D = 0.797, p = 0.043, BF10 = 2.22). No other stimulation conditions were different from the HC for reaction time (Fig. 5A and Table 5).
Within the HC participants, the reaction time was shorter during the long delay in comparison with the short delay (short delay = 0.674±0.209 s, long delay = 0.477±0.170 s, t(215) = – 9.51, Cohen’s D = 1.26, p < 0.001, BF10 > 50) (Fig. 5B and Table 6).
Movement time
There was no interaction between retention delay and STN-DBS condition for movement time (F(3, 731) = 0.29, p = 0.835). There was a main effect of STN-DBS condition for movement time (F(3, 731) = 12.92, p < 0.001). Post hoc analysis revealed that DBS-BOTH had a shorter movement time than DBS-RIGHT (DBS-BOTH = 0.973±0.262 s, DBS-RIGHT = 1.067±0.234 s, t(731) = – 4.04, Cohen’s D = 0.479, p < 0.001, BF10 > 50) and DBS-OFF (DBS-BOTH = 0.973±0.262 s, DBS-OFF = 1.097±0.271 s, t(731) = – 5.49, Cohen’s D = 0.564, p < 0.001, BF10 > 50). DBS-LEFT had a shorter movement time than DBS-RIGHT (DBS-LEFT = 1.000±0.253 s, DBS-RIGHT = 1.067±0.234 s, t(731) = – 2.94, Cohen’s D = 0.378, p = 0.018, BF10 = 13.1) and DBS-OFF (DBS-LEFT = 1.000±0.253 s, DBS-OFF = 1.097±0.271 s, t(731) = – 4.36, Cohen’s D = 0.470, p < 0.001, BF10 > 50). No other conditions were different from each other (Fig. 6A).
Fig. 6
A) Estimated mean (±standard error) of movement time for DBS-OFF, RIGHT, LEFT, BOTH, and the HC (left to right). DBS-LEFT and DBS-BOTH decreased movement time relative to DBS-OFF (p < 0.001 for both). DBS-BOTH and DBS-LEFT also decreased movement time relative to DBS-RIGHT (p < 0.001 and p = 0.018, respectively). The bars above the graph denote the significant differences in movement time between STN-DBS conditions. B) The effect of retention delay on movement time in the participants with PD and HC. Movement time was significantly decreased during the 0.5 s delay relative to the 5 s delay in participants with PD and HC (p < 0.001 and p = 0.001, respectively). The bars above the graph denote the significant differences in movement time between the short and long retention delays.
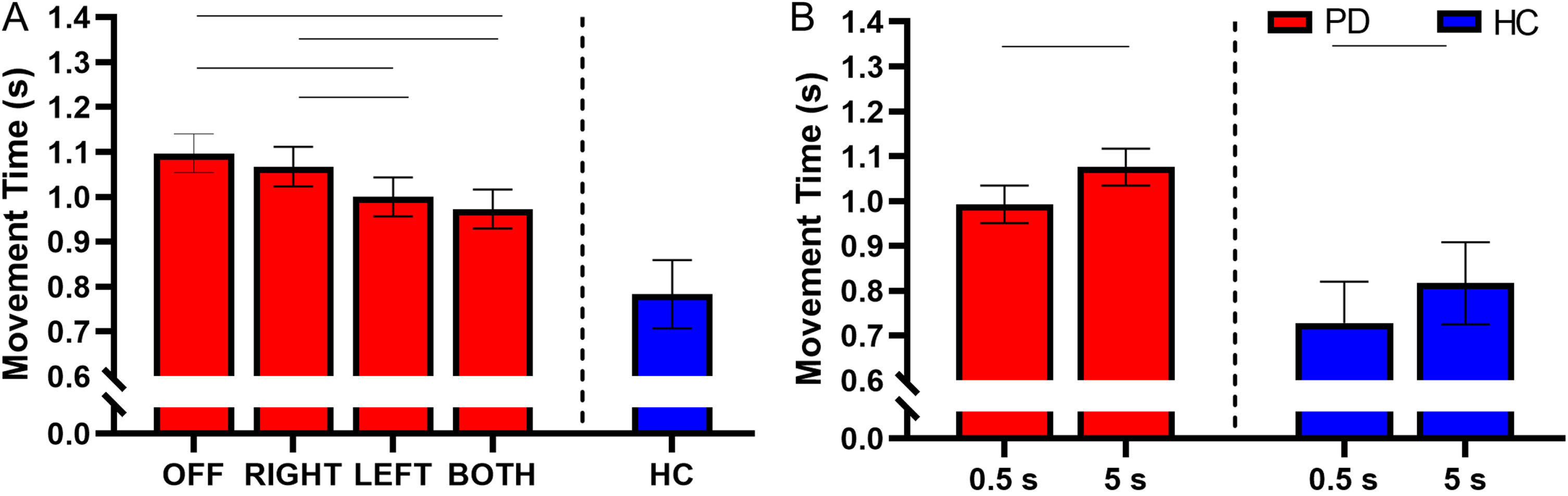
There was a main effect of retention delay on movement time in participants with PD. Movement time was shorter during the short delay in comparison to the long delay (F(1, 731) = 27.07, short delay = 0.993±0.241 s, long delay = 1.076±0.268 s, Cohen’s D = 0.445, p < 0.001, BF10 > 50) (Fig. 6B). For a full list of statistical values, see Table 4.
Compared with the HC, the participants with PD had significantly longer movement times in the DBS-LEFT (HC = 0.783±0.361 s, DBS-LEFT = 1.008±0.253 s, t(413) = 2.07, Cohen’s D = 0.816, p = 0.039, BF10 = 3.42), DBS-RIGHT (HC = 0.783±0.361 s, DBS-RIGHT = 1.068±0.234 s, t(377) = 2.55, Cohen’s D = 1.027, p = 0.011, BF10 = 7.37), and DBS-OFF (HC = 0.783±0.361 s, DBS-OFF = 1.100±0.271 s, t(385) = 2.77, Cohen’s D = 1.104, p = 0.006, BF10 = 14.35) conditions (Fig. 6A and Table 5). The difference between DBS-BOTH and HC did not reach significance (HC = 0.783±0.361 s, DBS-BOTH = 0.983±0.262 s, t(395) = 1.78, Cohen’s D = 0.671, p = 0.075, BF10 = 1.72).
Within the HC participants, the movement time was shorter during the short delay in comparison with the long delay (short delay = 0.728±0.311 s, long delay = 0.817±0.397 s, t(215) = 3.53, Cohen’s D = 0.870, p = 0.001, BF10 = 17.84) (Fig. 6B and Table 6).
DISCUSSION
Our results support our hypothesis and illustrated that both unilateral and bilateral STN-DBS improved intensive aspects of movement (amplitude and velocity) while impairing coordinative aspects of movement (error) during a memory-guided reaching task in which the retention delay was manipulated. Reaction time, in contrast, was unaffected by STN-DBS, and movement time was decreased by STN-DBS. In addition, the improvements in movement amplitude, movement velocity, and movement time were in the direction towards HC, while the impairment in error was in the direction away from HC. Furthermore, we found that a shorter retention delay resulted in increased amplitude and velocity of movement, smaller error, and decreased movement time, but also contributed to longer reaction times in the participants with PD, which was similar to what was seen in HC. Finally, there was no interaction between STN-DBS condition and retention delay for any of the outcome measures. Overall, these results support the notion that STN-DBS has differential effects on intensive and coordinative aspects of movement. Below we discuss the implications of these findings in further detail.
STN-DBS improves intensive but impairs coordinative aspects of movement
STN-DBS improved reaching amplitude and velocity toward the direction of HC, but also increased reaching error in the direction away from HC. These findings, along with our other results, were strengthened by calculation of Bayes Factors, which demonstrated that the alternative hypotheses were much more likely than our null hypotheses for each of our significant findings. The changes in intensive and coordinative aspects of motor control induced by STN-DBS may be resultant of alterations to the underlying physiology throughout the cortico-basal ganglia network, such as changes to the oscillatory activity driving movement, or alterations to the activity in regions connected with the STN that are implicated in movement planning and cognitive motor control. Prior to movement onset, there is a suppression of beta band oscillatory activity that is sustained throughout the movement [45–50]. In persons with PD, hyper-synchronization within the beta band is seen throughout the basal ganglia and between the sensorimotor cortices [20–22, 51]. Therefore, the increased beta synchronization in persons with PD may result in difficulties with peri-movement beta band suppression that is necessary for proper movement execution to occur [52], ultimately contributing to the decreased movement amplitude and velocity (i.e., intensive aspects of movement). STN-DBS effectively reduces the pathologically high beta band oscillatory activity within the STN in parallel to improvements in motor functioning [23–27]. Furthermore, previous work has illustrated that high frequency gamma band activity is reduced by STN-DBS, which in turn is associated with a reduction in motor symptoms in persons with PD [23]. Thus, these changes in beta and gamma oscillatory activity may be a physiological mechanism by which STN-DBS facilitates movement execution and improves intensive aspects of movement.
On the other hand, coordinative aspects of movement are more complex and require time-specific neural activations across various brain regions involved in sensorimotor integration, making them more difficult to manipulate properly with STN-DBS. STN-DBS can have detrimental effects on cognitive-motor tasks, especially when the cognitive demand is increased [14, 15, 53, 54]. STN-DBS can also alter activity within the delta and theta frequency bands within the prefrontal cortex [55]. Activity within the theta frequency band has been shown to underlie communication between distinct brain regions that regulate cognition [56–58]. It is possible that the modulation of these bands by STN-DBS disrupts the communication between the STN and cortex and contributes to the deficits in coordinative motor control [22]. Furthermore, alpha, beta, theta, and gamma oscillations are present while observing the actions of others, which may assist with motor learning [59, 60], suggesting that the modulation of these bands by STN-DBS may also contribute to impairment in coordinative motor control.
STN-DBS may also affect coordinative aspects of movement by antidromically altering activity within regions outside the STN that are involved in cognitive-motor control and movement planning. The movements performed during memory-guided reaching tasks are internally driven; they are driven by memory and not by an external stimulus. Regions such as the premotor cortex, dorsolateral prefrontal cortex, and supramarginal gyrus are implicated in the planning of internally driven movements [61]. Furthermore, the dorsolateral prefrontal cortex is active during coordinative tasks that are dependent on spatial working memory [62, 63]. Potentially, activity within these regions is altered by STN-DBS, adversely affecting movement planning and cognitive motor control. For example, the STN has direct connections with the dorsal premotor cortex [64], a cortical area essential for motor planning and integration of spatial coordinate systems between the eyes, hands, and the target of movement [65, 66]. Furthermore, STN-DBS has been shown to alter activity in the posterior parietal cortices [67–69], a region critically engaged with the integration of visual and motor information [70, 71]. Disruption to the activity within the dorsal premotor cortex and posterior parietal cortex may impair the integration of spatial coordinate systems and visuomotor transformations, adversely impacting effective planning of the ensuing movements and thereby increasing error.
Furthermore, STN-DBS may be adversely affecting the processes underlying spatial working memory in the participants with PD. People with PD have consistently illustrated deficiencies in working memory [34, 72–76]. If STN-DBS is disrupting the brain areas implicated in these processes, this disruption alone may be implicit in the detriment to coordinative aspects of movement performance. However, we think this is unlikely since David et al., 2018 previously found that the error of eye movements toward the targets during a sequential memory-guided reaching task was not disrupted by STN-DBS, suggesting that spatial working memory was not significantly affected by STN-DBS [16].
Reaction time was unaffected across all STN-DBS conditions. Previous literature has demonstrated inconsistent effects of STN-DBS on reaction time, with some evidence showing little or no effect [77], and other studies showing decreases in both simple and choice reaction time [9, 78]. Thus, the effects of STN-DBS on reaction time may be task specific. If STN-DBS is adversely impacting the planning of movement during the memory-guided reaching task, longer reaction times would be expected. However, STN-DBS also may decrease the time it takes to disinhibit movement execution. Thus, STN-DBS may have induced longer motor planning periods during the memory-guided reaching task but also facilitated movement onset, ultimately resulting in similar reaction times compared with STN-DBS off.
Finally, STN-DBS decreased movement time toward the direction of HC, similar to the findings of a previous study [7]. It is possible that the decreased movement time induced by STN-DBS may be a result of the increased velocity induced by STN-DBS. Increased movement time in older adults has been associated with increased levels of cortical beta band power, suggesting that an inability to suppress beta power during movement may result in a longer time to complete the movement [79]. As highlighted above, STN-DBS reduces beta band power, and this may ultimately facilitate a faster movement in people with PD.
Shorter retention delays increased velocity and amplitude, decreased error, and decreased movement times, but increased reaction times in people with PD
The trials that had a shorter retention delay (0.5 s) resulted in increased amplitude and velocity of movement, decreased movement times, and reduced error in the participants with PD. Despite this, a shorter retention period also significantly increased the reaction time. Interestingly, similar patterns were seen in the HC group, indicating that the mechanisms by which these aspects of movement are impacted by the retention delay are likely a result of physiological mechanisms that remain intact in participants with PD. For instance, the effect of retention delay on reaction time is a common physiological phenomenon [33, 80–82], in which long delays are thought to provide more time for movement planning prior to the cue, which saves time later [33]. In the current study, the 0.5 s window during the short delay trials was likely too brief to encode the target location and form the sensorimotor plan. Thus, when the cue to move was elicited, these processes may still have been ongoing, resulting in delayed movement and ultimately increased reaction time. At the same time, the longer retention delay likely results in more deterioration of the memorized spatial location of the target. This can lead to greater uncertainty about the target’s location, and this may have contributed to both a slower and less accurate reach.
The longer reaction times during the short delay corresponded with shorter movement times, an effect that has been illustrated before and may be a result of the context of the movement [83]. For instance, during stop-signal tasks, on trials in which there is a possibility of a stop signal, participants have longer reaction times, allowing for better coding of the movement and shorter movement times. Alternatively, shorter reaction times do not allow efficient coding of the movement. Therefore, the movement plan must be completed during the execution of the movement, which requires proactive inhibitory control that leads to longer movement times [83]. Thus, in our memory-guided reaching task, the longer reaction times seen during the short delay may have allowed participants to completely code the movement, ultimately resulting in decreased movement times.
Importantly, there were no interactions between STN-DBS condition and retention delay for any of the outcome measures. Presumably, if STN-DBS was affecting the ability to retain the target location in memory, there would have been a differential effect of delay based on condition (e.g., DBS-BOTH would produce a larger difference in error between the short and long retention delays in comparison with DBS-OFF). This further supports the notion that STN-DBS did not affect the spatial working memory components of the task and, specifically, STN-DBS did not disrupt the retention of the encoded target in memory.
Bilateral STN-DBS had a greater effect than unilateral STN-DBS on memory-guided reaching performance
Bilateral STN-DBS resulted in more significant improvements to intensive aspects of movement than unilateral STN-DBS. As discussed above, STN-DBS may induce a reduction in beta band synchronization and increase in gamma band synchronization which could facilitate movement execution and increase the amplitude and velocity of movement. The peri-movement beta desynchronization occurs on both the ipsilateral and contralateral hemispheres during movement [50, 84]. Thus, bilateral STN-DBS may decrease the excessive beta band synchronization bilaterally, resulting in a greater increase in amplitude and velocity than stimulation on one side only. Simultaneously, bilateral STN-DBS resulted in greater detriment to coordinative aspects of movement than unilateral STN-DBS (i.e., error). The above-mentioned mechanisms by which STN-DBS may impair coordinative aspects of movement (altering delta and theta band activity or altering activity in areas implicated in cognitive motor control and movement planning) may be exacerbated when the stimulation is bilateral. Alternatively, the regions involved in coordinative movement control on the ipsilateral side of stimulation may compensate for this disruption during unilateral stimulation [16]. Interestingly, it should be noted that only bilateral and not unilateral STN-DBS impacts motor inhibition [85], reinforcing the notion that unilateral and bilateral STN-DBS may have distinct physiological effects within the brain.
Finally, we found that unilateral DBS of the left STN significantly increased amplitude, velocity, and error, but DBS of the right STN did not. This is likely because the task was performed with the right hand for each participant, and the STN primarily modulates activity within the motor cortex on the ipsilateral side of the brain. Thus, DBS of the left STN likely modulated the activity of the left motor cortex, which controlled the right upper limb used to perform the task, resulting in the more prominent effects on motor control compared with the stimulation of the right STN.
Limitations
Before closing, it is worthwhile to note several limitations of the current study. First, the participants with PD were all male. While some studies have indicated that STN-DBS has similar efficacy in treating motor and cognitive signs in both males and females with PD [86, 87], other studies have demonstrated slightly better efficacy in males [88–90]. Thus, the way in which STN-DBS affects motor and cognitive symptoms in males versus females is not well-established, and future research should explore whether the effects of STN-DBS and retention delay on memory-guided reaching performance differ based on sex.
Second, the total number of trials performed for each participant was low (10 trials per condition). However, it has been illustrated that performance stability in reaching kinematics occurs between 2.65 and 6.16 trials [91]. Thus, increasing the number of trials would be unlikely to change our results. Furthermore, performing additional trials within each condition may lead to participant fatigue.
Conclusions
We utilized a memory-guided reaching task to provide evidence verifying that STN-DBS improves intensive aspects of movement while impairing coordinative aspects of movement. These findings were substantiated by comparison with a HC group, in which the velocity and amplitude were increased, and movement time was decreased toward what was seen in the HC, whereas error was increased away from what was seen in the HC. We also demonstrated that a shorter retention delay results in increased velocity and amplitude, shortened movement time, and decreased error, but also increases the reaction time. Furthermore, we established that the effect of STN-DBS on intensive and coordinative aspects of movement were independent of retention delay. This suggests it is unlikely that STN-DBS impairs coordinative motor control by disrupting working memory retention, but rather affects the activity within the cortico-basal ganglia loop subserving the planning of movement and cognitive-motor control. Overall, these findings help infer the underlying neurophysiological processes affected by STN-DBS. Future work can expand on these findings by utilizing neuroimaging to determine whether task-related changes in oscillatory activity of the sensorimotor cortices during a memory-guided reaching task are associated with changes to the intensive and coordinative aspects of movement. Furthermore, utilizing memory-guided reaching tasks to test the effectiveness of STN-DBS under different stimulation parameters may pave the way for establishing the most effective STN-DBS parameters that optimize both intensive and coordinative aspects of movement control.
ACKNOWLEDGMENTS
This study was supported by National Institutes of Health (R56 NS040902, R01 NS09295001, F31 NS12069501, T32 NS047987, and T32 HD07418).
CONFLICT OF INTEREST
Authors FD, MM, and MT received grant support from the NIH. Author SS received grant support from the NIH, Medtronic, Abbott, Boston Scientific, and consults for Insightec. Author JR has consulting contracts with Stryker, Boston Scientific, Neuromodulation, Monteris, and AIM Medical Robotics. Author GP received grant support from the NIH and consults for Guidepoint and Kyowa Kirin. Author LV M receives honoraria for consulting services/advisory boards from AbbVie, Abbott, Avion, and research support from Abbott, Boston Scientific, Medtronic, Neuroderm, and NIH. Author DC has stock ownership in Medtronic, consultancies with University of Illinois Chicago and Council for Jewish Elderly SeniorLife, honoraria from MDS, 2021 Shanghai International Symposium on Medicine in the 21st Century, ACSM, Marianjoy Rehabilitation Hospital, and MDS-PAS 2022, received grant support from the NIH and Michael J. Fox foundation, and receives lecture and reviewer fees from NIH.
No other authors have conflicts of interest to report.
DATA AVAILABILITY
The data supporting the findings of this study are available upon reasonable request to the corresponding author.
REFERENCES
[1] | Dorsey ER , Constantinescu R , Thompson JP , Biglan KM , Holloway RG , Kieburtz K , Marshall FJ , Ravina BM , Schifitto G , Siderowf A , Tanner CM ((2007) ) Projected number of people with Parkinson disease in the most populous nations, 2005 through 2030. Neurology 68: , 384–386. |
[2] | de Lau LM , Breteler MM ((2006) ) Epidemiology of Parkinson’s disease. Lancet Neurol 5: , 525–535. |
[3] | Tysnes OB , Storstein A ((2017) ) Epidemiology of Parkinson’s disease. J Neural Transm (Vienna) 124: , 901–905. |
[4] | Vaillancourt DE , Prodoehl J , Verhagen Metman L , Bakay RA , Corcos DM ((2004) ) Effects of deep brain stimulation and medication on bradykinesia and muscle activation in Parkinson’s disease. Brain 127: , 491–504. |
[5] | Vaillancourt DE , Prodoehl J , Sturman MM , Bakay RA , Metman LV , Corcos DM ((2006) ) Effects of deep brain stimulation and medication on strength, bradykinesia, and electromyographic patterns of the ankle joint in Parkinson’s disease. Mov Disord 21: , 50–58. |
[6] | Mirabella G , Iaconelli S , Romanelli P , Modugno N , Lena F , Manfredi M , Cantore G ((2012) ) Deep brain stimulation of subthalamic nuclei affects arm response inhibition in Parkinson’s patients. Cereb Cortex 22: , 1124–1132. |
[7] | Mirabella G , Iaconelli S , Modugno N , Giannini G , Lena F , Cantore G ((2013) ) Stimulation of subthalamic nuclei restores a near normal planning strategy in Parkinson’s patients. PLoS One 8: , e62793. |
[8] | Swann N , Poizner H , Houser M , Gould S , Greenhouse I , Cai W , Strunk J , George J , Aron AR ((2011) ) Deep brain stimulation of the subthalamic nucleus alters the cortical profile of response inhibition in the beta frequency band: A scalp EEG study in Parkinson’s disease. J Neurosci 31: , 5721–5729. |
[9] | van den Wildenberg WP , van Boxtel GJ , van der Molen MW , Bosch DA , Speelman JD , Brunia CH ((2006) ) Stimulation of the subthalamic region facilitates the selection and inhibition of motor responses in Parkinson’s disease. J Cogn Neurosci 18: , 626–636. |
[10] | van Wouwe NC , Pallavaram S , Phibbs FT , Martinez-Ramirez D , Neimat JS , Dawant BM , D’Haese PF , Kanoff KE , van den Wildenberg WPM , Okun MS , Wylie SA ((2017) ) Focused stimulation of dorsal subthalamic nucleus improves reactive inhibitory control of action impulses. Neuropsychologia 99: , 37–47. |
[11] | Jahanshahi M ((2013) ) Effects of deep brain stimulation of the subthalamic nucleus on inhibitory and executive control over prepotent responses in Parkinson’s disease. Front Syst Neurosci 7: , 118. |
[12] | Goelz LC , David FJ , Sweeney JA , Vaillancourt DE , Poizner H , Metman LV , Corcos DM ((2017) ) The effects of unilateral versus bilateral subthalamic nucleus deep brain stimulation on prosaccades and antisaccades in Parkinson’s disease. Exp Brain Res 235: , 615–626. |
[13] | Munoz MJ , Goelz LC , Pal GD , Karl JA , Verhagen Metman L , Sani S , Rosenow JM , Ciolino JD , Kurani AS , Corcos DM , David FJ ((2022) ) Increased subthalamic nucleus deep brain stimulation amplitude impairs inhibitory control of eye movements in Parkinson’s disease. Neuromodulation 25: , 866–876. |
[14] | Alberts JL , Voelcker-Rehage C , Hallahan K , Vitek M , Bamzai R , Vitek JL ((2008) ) Bilateral subthalamic stimulation impairs cognitive-motor performance in Parkinson’s disease patients. Brain 131: , 3348–3360. |
[15] | Hershey T , Wu J , Weaver PM , Perantie DC , Karimi M , Tabbal SD , Perlmutter JS ((2008) ) Unilateral vs. bilateral STN DBS effects on working memory and motor function in Parkinson disease. Exp Neurol 210: , 402–408. |
[16] | David FJ , Goelz LC , Tangonan RZ , Metman LV , Corcos DM ((2018) ) Bilateral deep brain stimulation of the subthalamic nucleus increases pointing error during memory-guided sequential reaching. Exp Brain Res 236: , 1053–1065. |
[17] | Snider J , Lee D , Harrington DL , Poizner H ((2014) ) Scaling and coordination deficits during dynamic object manipulation in Parkinson’s disease. J Neurophysiol 112: , 300–315. |
[18] | Lukos JR , Snider J , Hernandez ME , Tunik E , Hillyard S , Poizner H ((2013) ) Parkinson’s disease patients show impaired corrective grasp control and eye-hand coupling when reaching to grasp virtual objects. Neuroscience 254: , 205–221. |
[19] | Schettino LF , Adamovich SV , Hening W , Tunik E , Sage J , Poizner H ((2006) ) Hand preshaping in Parkinson’s disease: Effects of visual feedback and medication state. Exp Brain Res 168: , 186–202. |
[20] | Cassidy M , Mazzone P , Oliviero A , Insola A , Tonali P , Di Lazzaro V , Brown P ((2002) ) Movement-related changes in synchronization in the human basal ganglia. Brain 125: , 1235–1246. |
[21] | Hammond C , Bergman H , Brown P ((2007) ) Pathological synchronization in Parkinson’s disease: Networks, models and treatments. Trends Neurosci 30: , 357–364. |
[22] | David FJ , Munoz MJ , Corcos DM ((2020) ) The effect of STN DBS on modulating brain oscillations: Consequences for motor and cognitive behavior. Exp Brain Res 238: , 1659–1676. |
[23] | Cao CY , Zeng K , Li DY , Zhan SK , Li XL , Sun BM ((2017) ) Modulations on cortical oscillations by subthalamic deep brain stimulation in patients with Parkinson disease: A MEG study. Neurosci Lett 636: , 95–100. |
[24] | Müller EJ , Robinson PA ((2018) ) Suppression of parkinsonian beta oscillations by deep brain stimulation: Determination of effective protocols. Front Comput Neurosci 12: , 98. |
[25] | Eusebio A , Cagnan H , Brown P ((2012) ) Does suppression of oscillatory synchronisation mediate some of the therapeutic effects of DBS in patients with Parkinson’s disease? Front Integr Neurosci 6: , 47. |
[26] | Kühn AA , Kempf F , Brücke C , Gaynor Doyle L , Martinez-Torres I , Pogosyan A , Trottenberg T , Kupsch A , Schneider GH , Hariz MI , Vandenberghe W , Nuttin B , Brown P ((2008) ) High-frequency stimulation of the subthalamic nucleus suppresses oscillatory beta activity in patients with Parkinson’s disease in parallel with improvement in motor performance. J Neurosci 28: , 6165–6173. |
[27] | Whitmer D , de Solages C , Hill B , Yu H , Henderson JM , Bronte-Stewart H ((2012) ) High frequency deep brain stimulation attenuates subthalamic and cortical rhythms in Parkinson’s disease. Front Hum Neurosci 6: , 155. |
[28] | Neumann WJ , Schroll H , de Almeida Marcelino AL , Horn A , Ewert S , Irmen F , Krause P , Schneider GH , Hamker F , Kühn AA ((2018) ) Functional segregation of basal ganglia pathways in Parkinson’s disease. Brain 141: , 2655–2669. |
[29] | Zavala BA , Jang AI , Zaghloul KA ((2017) ) Human subthalamic nucleus activity during non-motor decision making. Elife 6: , e31007. |
[30] | Medina J , Jax SA , Coslett HB ((2009) ) Two-component models of reaching: Evidence from deafferentation in a Fitts’ law task. Neurosci Lett 451: , 222–226. |
[31] | Sarlegna FR , Sainburg RL ((2009) ) The roles of vision and proprioception in the planning of reaching movements. Adv Exp Med Biol 629: , 317–335. |
[32] | Park J ((2005) ) Effect of arousal and retention delay on memory: A meta-analysis. Psychol Rep 97: , 339–355. |
[33] | Churchland MM , Shenoy KV ((2007) ) Delay of movement caused by disruption of cortical preparatory activity. J Neurophysiol 97: , 348–359. |
[34] | Ketcham CJ , Hodgson TL , Kennard C , Stelmach GE ((2003) ) Memory-motor transformations are impaired in Parkinson’s disease. Exp Brain Res 149: , 30–39. |
[35] | Hughes AJ , Daniel SE , Kilford L , Lees AJ ((1992) ) Accuracy of clinical diagnosis of idiopathic Parkinson’s disease: A clinico-pathological study of 100 cases. J Neurol Neurosurg Psychiatry 55: , 181–184. |
[36] | Hughes AJ , Ben-Shlomo Y , Daniel SE , Lees AJ ((1992) ) What features improve the accuracy of clinical diagnosis in Parkinson’s disease: A clinicopathologic study. Neurology 42: , 1142–1146. |
[37] | Simuni T , Caspell-Garcia C , Coffey CS , Weintraub D , Mollenhauer B , Lasch S , Tanner CM , Jennings D , Kieburtz K , Chahine LM , Marek K ((2018) ) Baseline prevalence and longitudinal evolution of non-motor symptoms in early Parkinson’s disease: The PPMI cohort. J Neurol Neurosurg Psychiatry 89: , 78–88. |
[38] | Carson N , Leach L , Murphy KJ ((2018) ) A re-examination of Montreal Cognitive Assessment (MoCA) cutoff scores. Int J Geriatr Psychiatry 33: , 379–388. |
[39] | Oldfield RC ((1971) ) The assessment and analysis of handedness: The Edinburgh inventory. Neuropsychologia 9: , 97–113. |
[40] | Temperli P , Ghika J , Villemure JG , Burkhard PR , Bogousslavsky J , Vingerhoets FJ ((2003) ) How do parkinsonian signs return after discontinuation of subthalamic DBS? Neurology 60: , 78–81. |
[41] | Goetz CG , Tilley BC , Shaftman SR , Stebbins GT , Fahn S , Martinez-Martin P , Poewe W , Sampaio C , Stern MB , Dodel R , Dubois B , Holloway R , Jankovic J , Kulisevsky J , Lang AE , Lees A , Leurgans S , LeWitt PA , Nyenhuis D , Olanow CW , Rascol O , Schrag A , Teresi JA , van Hilten JJ , LaPelle N ((2008) ) Movement Disorder Society-sponsored revision of the Unified Parkinson’s Disease Rating Scale (MDS-UPDRS): Scale presentation and clinimetric testing results. Mov Disord 23: , 2129–2170. |
[42] | Kelly VE , Hyngstrom AS , Rundle MM , Bastian AJ ((2002) ) Interaction of levodopa and cues on voluntary reaching in Parkinson’s disease. Mov Disord 17: , 38–44. |
[43] | Corcos DM , Gottlieb GL , Agarwal GC ((1989) ) Organizing principles for single-joint movements. II. A speed-sensitive strategy. J Neurophysiol 62: , 358–368. |
[44] | Fitts PM , Radford BK ((1966) ) Information capacity of discrete motor responses under different cognitive sets. J Exp Psychol 71: , 475–482. |
[45] | Cheyne D , Bakhtazad L , Gaetz W ((2006) ) Spatiotemporal mapping of cortical activity accompanying voluntary movements using an event-related beamforming approach. Hum Brain Mapp 27: , 213–229. |
[46] | Gaetz W , Macdonald M , Cheyne D , Snead OC ((2010) ) Neuromagnetic imaging of movement-related cortical oscillations in children and adults: Age predicts post-movement beta rebound. Neuroimage 51: , 792–807. |
[47] | Heinrichs-Graham E , Wilson TW ((2015) ) Coding complexity in the human motor circuit. Hum Brain Mapp 36: , 5155–5167. |
[48] | Jurkiewicz MT , Gaetz WC , Bostan AC , Cheyne D ((2006) ) Post-movement beta rebound is generated in motor cortex: Evidence from neuromagnetic recordings. Neuroimage 32: , 1281–1289. |
[49] | Pfurtscheller G , Lopes da Silva FH ((1999) ) Event-related EEG/MEG synchronization and desynchronization: Basic principles. Clin Neurophysiol 110: , 1842–1857. |
[50] | Wilson TW , Slason E , Asherin R , Kronberg E , Reite ML , Teale PD , Rojas DC ((2010) ) An extended motor network generates beta and gamma oscillatory perturbations during development. Brain Cogn 73: , 75–84. |
[51] | Heinrichs-Graham E , Kurz MJ , Becker KM , Santamaria PM , Gendelman HE , Wilson TW ((2014) ) Hypersynchrony despite pathologically reduced beta oscillations in patients with Parkinson’s disease: A pharmaco-magnetoencephalography study. J Neurophysiol 112: , 1739–1747. |
[52] | Heinrichs-Graham E , Wilson TW , Santamaria PM , Heithoff SK , Torres-Russotto D , Hutter-Saunders JA , Estes KA , Meza JL , Mosley RL , Gendelman HE ((2014) ) Neuromagnetic evidence of abnormal movement-related beta desynchronization in Parkinson’s disease. Cereb Cortex 24: , 2669–2678. |
[53] | Georgiev D , Dirnberger G , Wilkinson L , Limousin P , Jahanshahi M ((2016) ) In Parkinson’s disease on a probabilistic Go/NoGo task deep brain stimulation of the subthalamic nucleus only interferes with withholding of the most prepotent responses. Exp Brain Res 234: , 1133–1143. |
[54] | Williams IA , Wilkinson L , Limousin P , Jahanshahi M ((2015) ) Load-dependent interference of deep brain stimulation of the subthalamic nucleus with switching from automatic to controlled processing during random number generation in Parkinson’s disease. J Parkinsons Dis 5: , 321–331. |
[55] | Cavanagh JF , Wiecki TV , Cohen MX , Figueroa CM , Samanta J , Sherman SJ , Frank MJ ((2011) ) Subthalamic nucleus stimulation reverses mediofrontal influence over decision threshold. Nat Neurosci 14: , 1462–1467. |
[56] | Lam J , Lee J , Williams M , Cohn M , Wilson M , Mark C , Esnaashari N , Petkus A , Hui J , Feigenbaum D , Liker M , Liu CY , Lee B , Lee DJ ((2021) ) Cognitive effects of theta frequency bilateral subthalamic nucleus stimulation in Parkinson’s disease: A pilot study. Brain Stimul 14: , 230–240. |
[57] | Berens SC , Horner AJ ((2017) ) Theta rhythm: Temporal glue for episodic memory. Curr Biol 27: , R1110–r1112. |
[58] | Buzsáki G , Moser EI ((2013) ) Memory, navigation and theta rhythm in the hippocampal-entorhinal system. Nat Neurosci 16: , 130–138. |
[59] | Babiloni C , Del Percio C , Vecchio F , Sebastiano F , Di Gennaro G , Quarato PP , Morace R , Pavone L , Soricelli A , Noce G , Esposito V , Rossini PM , Gallese V , Mirabella G ((2016) ) Alpha, beta and gamma electrocorticographic rhythms in somatosensory, motor, premotor and prefrontal cortical areas differ in movement execution and observation in humans. Clin Neurophysiol 127: , 641–654. |
[60] | Babiloni C , Del Percio C , Lopez S , Di Gennaro G , Quarato PP , Pavone L , Morace R , Soricelli A , Noce G , Esposito V , Gallese V , Mirabella G ((2017) ) Frontal functional connectivity of electrocorticographic delta and theta rhythms during action execution versus action observation in humans. Front Behav Neurosci 11: , 20. |
[61] | Ariani G , Wurm MF , Lingnau A ((2015) ) Decoding internally and externally driven movement plans. J Neurosci 35: , 14160–14171. |
[62] | Barbey AK , Koenigs M , Grafman J ((2013) ) Dorsolateral prefrontal contributions to human working memory. Cortex 49: , 1195–1205. |
[63] | Owen AM , McMillan KM , Laird AR , Bullmore E ((2005) ) N-back working memory paradigm: A meta-analysis of normative functional neuroimaging studies. Hum Brain Mapp 25: , 46–59. |
[64] | Nambu A , Tokuno H , Inase M , Takada M ((1997) ) Corticosubthalamic input zones from forelimb representations of the dorsal and ventral divisions of the premotor cortex in the macaque monkey: Comparison with the input zones from the primary motor cortex and the supplementary motor area. Neurosci Lett 239: , 13–16. |
[65] | Graziano MS ((2006) ) Progress in understanding spatial coordinate systems in the primate brain. Neuron 51: , 7–9. |
[66] | Pesaran B , Nelson MJ , Andersen RA ((2006) ) Dorsal premotor neurons encode the relative position of the hand, eye, and goal during reach planning. Neuron 51: , 125–134. |
[67] | Hilker R , Voges J , Weisenbach S , Kalbe E , Burghaus L , Ghaemi M , Lehrke R , Koulousakis A , Herholz K , Sturm V , Heiss WD ((2004) ) Subthalamic nucleus stimulation restores glucose metabolism in associative and limbic cortices and in cerebellum: Evidence from a FDG-PET study in advanced Parkinson’s disease. J Cereb Blood Flow Metab 24: , 7–16. |
[68] | Trost M , Su S , Su P , Yen RF , Tseng HM , Barnes A , Ma Y , Eidelberg D ((2006) ) Network modulation by the subthalamic nucleus in the treatment of Parkinson’s disease. Neuroimage 31: , 301–307. |
[69] | Vafaee MS , ØStergaard K , Sunde N , Gjedde A , Dupont E , Cumming P ((2004) ) Focal changes of oxygen consumption in cerebral cortex of patients with Parkinson’s disease during subthalamic stimulation. Neuroimage 22: , 966–974. |
[70] | Batista AP , Buneo CA , Snyder LH , Andersen RA ((1999) ) Reach plans in eye-centered coordinates. Science 285: , 257–260. |
[71] | Buneo CA , Jarvis MR , Batista AP , Andersen RA ((2002) ) Direct visuomotor transformations for reaching. Nature 416: , 632–636. |
[72] | Fournet N , Moreaud O , Roulin JL , Naegele B , Pellat J ((2000) ) Working memory functioning in medicated Parkinson’s disease patients and the effect of withdrawal of dopaminergic medication. Neuropsychology 14: , 247–253. |
[73] | Bradley VA , Welch JL , Dick DJ ((1989) ) Visuospatial working memory in Parkinson’s disease. J Neurol Neurosurg Psychiatry 52: , 1228–1235. |
[74] | Morris RG , Downes JJ , Sahakian BJ , Evenden JL , Heald A , Robbins TW ((1988) ) Planning and spatial working memory in Parkinson’s disease. J Neurol Neurosurg Psychiatry 51: , 757–766. |
[75] | Helmuth LL , Mayr U , Daum I ((2000) ) Sequence learning in Parkinson’s disease: A comparison of spatial-attention and number-response sequences. Neuropsychologia 38: , 1443–1451. |
[76] | Seidler RD , Tuite P , Ashe J ((2007) ) Selective impairments in implicit learning in Parkinson’s disease. Brain Res 1137: , 104–110. |
[77] | Nakamura K , Christine CW , Starr PA , Marks WJ Jr. , ((2007) ) Effects of unilateral subthalamic and pallidal deep brain stimulation on fine motor functions in Parkinson’s disease. Mov Disord 22: , 619–626. |
[78] | Kumru H , Summerfield C , Valldeoriola F , Valls-Solé J ((2004) ) Effects of subthalamic nucleus stimulation on characteristics of EMG activity underlying reaction time in Parkinson’s disease. Mov Disord 19: , 94–100. |
[79] | Heinrichs-Graham E , Wilson TW ((2016) ) Is an absolute level of cortical beta suppression required for proper movement? Magnetoencephalographic evidence from healthy aging. Neuroimage 134: , 514–521. |
[80] | Crammond DJ , Kalaska JF ((2000) ) Prior information in motor and premotor cortex: Activity during the delay period and effect on pre-movement activity. J Neurophysiol 84: , 986–1005. |
[81] | Riehle A , MacKay WA , Requin J ((1994) ) Are extent and force independent movement parameters? Preparation- and movement-related neuronal activity in the monkey cortex. Exp Brain Res 99: , 56–74. |
[82] | Rosenbaum DA ((1980) ) Human movement initiation: Specification of arm, direction, and extent. J Exp Psychol Gen 109: , 444–474. |
[83] | Mirabella G , Pani P , Ferraina S ((2008) ) Context influences on the preparation and execution of reaching movements. Cogn Neuropsychol 25: , 996–1010. |
[84] | Wilson TW , Heinrichs-Graham E , Becker KM ((2014) ) Circadian modulation of motor-related beta oscillatory responses. Neuroimage 102: (Pt 2), 531–539. |
[85] | Mancini C , Modugno N , Santilli M , Pavone L , Grillea G , Morace R , Mirabella G ((2018) ) Unilateral stimulation of subthalamic nucleus does not affect inhibitory control. Front Neurol 9: , 1149. |
[86] | Kim R , Yoo D , Choi JH , Shin JH , Park S , Kim HJ , Paek SH , Jeon B ((2019) ) Sex differences in the short-term and long-term effects of subthalamic nucleus stimulation in Parkinson’s disease. Parkinsonism Relat Disord 68: , 73–78. |
[87] | Chandran S , Krishnan S , Rao RM , Sarma SG , Sarma PS , Kishore A ((2014) ) Gender influence on selection and outcome of deep brain stimulation for Parkinson’s disease. Ann Indian Acad Neurol 17: , 66–70. |
[88] | Yuan TS , Chen YC , Liu DF , Ma RY , Zhang X , Du TT , Zhu GY , Zhang JG ((2023) ) Sex modulates the outcome of subthalamic nucleus deep brain stimulation in patients with Parkinson’s disease. Neural Regen Res 18: , 901–907. |
[89] | Accolla E , Caputo E , Cogiamanian F , Tamma F , Mrakic-Sposta S , Marceglia S , Egidi M , Rampini P , Locatelli M , Priori A ((2007) ) Gender differences in patients with Parkinson’s disease treated with subthalamic deep brain stimulation. Mov Disord 22: , 1150–1156. |
[90] | Romito LM , Contarino FM , Albanese A ((2010) ) Transient gender-related effects in Parkinson’s disease patients with subthalamic stimulation. J Neurol 257: , 603–608. |
[91] | Chen Y , Garcia-Vergara S , Howard A ((2020) ) Number of trials necessary to achieve performance stability in a reaching kinematics movement analysis game. J Hand Ther 33: , 371–377.e371. |




